|
PAGE |
QUOTEs
(Most quotes verbatim Henri Louis
Bergson, some paraphrased.) |
COMMENTs
(Relevant to Pirsig, William James
Sidis, and Quantonics Thinking Modes.) |
|
117 |
| "A direct analysis of the notion
of velocity will bring us to the same conclusion.
Mechanics gets |
| This is seen is the definition of velocity. |
this notion through a series of ideas, the connexion
of which it is easy enough to trace. It first |
builds up the idea of uniform motion by picturing, on
the one hand, the path AB of a certain moving body, and, on the
other, a physical phenomenon which is repeated indefinitely under
the same conditions, e.g., a stone always falling from the same
height on to the same spot. If we mark on the path AB the points
M, N, P . . . reached by the moving body at each of the moments
when the stone touches the ground, and if the intervals AM, MN
and NP are found to be equal to one another, the motion will
be said to be uniform: and any one of these intervals
will be called the velocity
of the moving body, provided that it is agreed to adopt as unit
of duration the physical phenomenon which has been chosen as
the term of comparison. Thus, the velocity
of a uniform motion is defined by mechanics
without appealing to any other notions than those of space and
simultaneity." |
(Our bold and color.)
Bergson restarts his footnote counts on each page. So to refer
a footnote, one must state page number and footnote number.
Our bold and color highlights follow a code:
- black-bold - important to read if you are just scanning
our review
- orange-bold - text ref'd
by index pages
- green-bold - we see Bergson
suggesting axiomatic memes
- violet-bold - an apparent
classical problematic
- blue-bold - we disagree
with this text segment while disregarding context of Bergson's
overall text
- gray-bold - quotable
text
- red-bold - our direct
commentary
|
| 118 |
"Now let us turn to the case
of a variable motion, that is, to the case when the elements
AM, MN, NP . . . are found to be unequal. In order to define
the velocity of the moving body A at the point M, we shall only
have to imagine an unlimited number of moving bodies A1,
A2, A3 . .all moving uniformly with velocities
v1, v2, v3
. . . which are arranged, e.g., in an ascending scale and
which correspond to all possible magnitudes. Let us then consider
on the path of the moving body A two points M' and M", situated
on either side of the point M but very near it. At the same time
as this moving body reaches the points M', M, M", the other
moving bodies reach points M'1, M1, M"1,
M'2 M2, M"2 . . . on their
respective paths; and there must be two moving bodies Ah
and Ap, such that we have on the one hand M'M = M'hMh,
and on the other hand MM" = MpM"p.
We shall then agree to say that the velocity of the moving body
A at the point M lies between vh and vp.
But nothing prevents our assuming that the points M' and M"
are still nearer the point M, and it will then be necessary to
replace vh and vp, by two
fresh velocities vi, and vn,
the one greater than vh, and the other less
than vp. And in proportion as we reduce the
two intervals M'M and MM", we shall lessen the difference
between the velocities of
the uniform corresponding movements." |
(Our bold and color.)
Bergson introduces a simple spatial calculus of velocity.
Perhaps most important observation to make about pages 117
and 118 is that Bergson requires no notion of time to express
a classical concept of velocity. We may choose to view this as
a quantum tell that some specific meme more fundamental than
mass, length, and time underlies those classical indefinables.
In Quantonics we call it "flux." You may then choose
to view Bergson's finite, yet variable, analytic decomposition
of linear space as a proxy for flux just as Irving
Stein does in his one-dimensional "quantum object"
models. Doug - 23May2002.
Return to Chapter Index
|
| 119 |
"Now, the two intervals being
capable of decreasing right down to zero, there evidently exists
between vi and vn a certain velocity
vm, such that the difference between this velocity
and vh, vi. . . on the one
hand, and vp, vn. . . on
the other, can become smaller than any given quantity. It is
this common limit vm which we shall call the
velocity of the moving body A at the point [point
is an extremely problematic classical concept; infinitesimal
'points' do n¤t 'exist' in quantum reality] M.—Now,
in this analysis of variable motion, as in that of uniform motion,
it is a question only of spaces once traversed and of simultaneous
positions once reached. We were thus justified in saying that,
while all that mechanics retains of
time is simultaneity, all
that it retains of motion itself—restricted, as it is, to a measurement
of motion—is
immobility.
| "This result
might have been foreseen by noticing that mechanics
necessarily deals with equations, |
| Mechanics
deals with equations, which express something finished,
and not processes, such as duration and
motion. |
and that an algebraic
equation always expresses something
already done. Now, it is of the very essence
of duration
and motion as they appear to our consciousness, to be something
that is unceasingly being done; thus algebra
can |
represent the results gained
at a certain moment of duration and the positions occupied by
a certain moving body in space, but [mathematical formulae can-] not [represent] duration and motion
themselves. Mathematics may, indeed, increase the number of simultaneities
and positions which it takes into consideration by making the
intervals very small: it may even, by using the differential instead of the difference,
show that it is possible to increase without limit the number
of these intervals of duration." |
(Our brackets, bold and color.)
In other words, formal mechanical (i.e., mathematical) analysis
of reality samples 'state' and classical 'state' is innately
(by classical axiom) state-ic, and thus "immobile."
Juxtapose this classical requisite with a quantum imperative
that reality is absolute changæ/flux.
Algebra is/expresses n¤nexistent
ESQ! Reality is, as Bergson
told us prior, animate — instable.
Duration is animate process
and as such algebraically n¤nanalyzable.
Quantum reality is both duration
and motion. Bergson tells us that classical mathematics has n¤
intrinsic means of representing quantum reality.
Bravo! We agree.
We also offer a heuristic that classical English language
is similarly, due its own analytic state-icity, incapable of
representing quantum reality and that is our motivation here
in Quantonics to Remediate English
Language for Millennium III.
Finally, and most important of all, we must learn to understand
that mass, length/space, and time are all quantum processes,
n¤t classical state-ic
'measurables.'
Return to Chapter Index
|
| 120 |
"Nevertheless, however small
the interval is supposed to be, it is
the extremity of the interval at which mathematics always places
itself. As for the interval itself, as for the duration and the
motion, they are necessarily left out of the equation.
The reason is that duration and motion
are mental syntheses, and not
objects; that, although the moving body occupies, one after the
other, points on a line, motion itself
has nothing
to do with a line; and finally that, although the
positions occupied by the moving body vary with the different
moments of duration, though it even creates distinct moments
by the mere fact of occupying different positions, duration
properly so called has no
moments which are identical or external to one another, being
essentially heterogeneous,
continuous, and with no analogy
to number.
| "It follows from this analysis that space alone [as a state-ic, immutable,
excluded-middle, infinitely divisible classical concept]
is homogeneous, that |
| Conclusion:
space
alone is homogeneous: duration and succession belong not to the external world,
but to the conscious mind. |
objects in space
form a discrete multiplicity, and that every discrete
multiplicity is got by a process of unfolding in space.
It also follows that there is neither
duration nor even succession in space, if we
give to these words the meaning in which consciousness takes
them: each of the so-called successive states
of the external world exists |
alone [i.e., classically lisr];
their multiplicity is real only for a consciousness that can
first retain them and then set them side by side by externalizing
them in relation to one another. If it retains them, it is because
these distinct states
of the external world give rise to states
of consciousness which permeate one
another, imperceptibly organize themselves into a
whole, and bind the past to the present by this very process
of connexion. If it externalizes them in relation [juxtaposition]
to one another, the reason is that, thinking of their radical
distinctness (the one having ceased to be when the other appears
on the scene), it perceives them under the form of a discrete
multiplicity, which amounts to setting them out in line, in the
space in which each of them
existed separately. The space employed for this purpose is just
that which is called homogeneous time."
[Crux! Classical time 'is' space 'is' homogeneous time!] |
(Our brackets, bold, color, and violet bold italic problematics.
Symbol font required for comments on this page.)
And, dear reader, only quantum computers can offer us real
analogies of our quantum stages' mental 'syntheses' of duration
and motion. Formal, radically mechanistic, classical, digital
computers never will! (Quantum computers have qubits vis-à-vis
classical computers have digits/bits/nats/etc. Where qubits are
quantons, digits are dichons:
- Qubits are quantumly l¤gical:
- heterogeneous,
 Planck
rate mutable, Planck
rate mutable,
 quantal, quantal,
- (per
intera) everywhere-associative via quantum/quantonic
ensemble interrelationships mediated by quantum vacuum flux,
 included-middle, included-middle,
 coquecigrues(ical) coquecigrues(ical)
- animate,
 phase-ic, phase-ic,
- pragmadigmatic,
- coobsfecting,
- (classically-) innumerable,
 stochastic,
(quanton_y stochastic,
(quanton_y f(flux)) f(flux))
- c¤mplementary,
- ensemble quantum
c¤mplementarity:
 qubit qubit

quantons(c¤mplementsn,quantons(c¤mplementself,c¤mplementsothers))
There are countless ways to show and hermeneut that script. We
tried to retain part of our initial description while advancing
n¤vel memes of l¤cal and n¤nl¤cal
EIMA fuzzonic
interrelationshipings.
New red text effaces ,c¤mplement1 which on
its face is monistically, monastically naïve.
Doug - 20Oct2006.
 ensemble
quantum umcærtainty, ensemble
quantum umcærtainty,
i.e.,
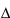 u1 u1 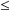
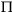 ( (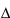 c¤mplement1· c¤mplement1· c¤mplementsn), c¤mplementsn),
where our plural use of "c¤mplements" represents
heterogeneity of other quantum c¤mplementsn
which have ensemble affective quantum umcærtainty
interrelationships with c¤mplement1, including
complement1's umcærtainty interrelationships
with itself. (Our use of classical analytic mathematics is inappropriate
here, and we do so only to bequeath a heretofore and yet wanting
semantic of real ensemble quantum umcærtainty.)
- omnifference,
- etc.
- Digits/bits/nats/etc. are classically logical:
- homogeneous,
- immutable,
- (per se, objective, denying both subjective reality
and quantum vacuum flux) lisr,
- excluded-middle,
- inanimate/stable,
- state-ic,
- paradigmatic,
- unilaterally observable (lisrable),
- predicate logically negatable,
- (classically-) numerable,
- determinate, (y=f(t))
- certain, (causation & 1-1 correspondence)
- difference,
- etc.)
|